Content
Kinematics: Radial Motion and Spherical Coordinates
Component Functions of
Spherical Coordinate System
Unit Vectors in Spherical Coordinates
Unit Vector
Derivatives in Spherical Coordinates
Position Vector in Spherical Coordinates
Velocity Vector in Spherical Coordinates
Acceleration Vector in Spherical Coordinates
Motion Functions in Spherical Coordinates
Kinematics: Radial Motion and Spherical Coordinates
In practical engineering problems, sometimes it is convenient to resolve the component functions of the vector function of motion into components which is the radial components of the object with respect to a reference origin of the motion together with the azimuthal and polar components of two anglular motions. Thus the study of the motion can be focused on the motion of an object along the radial direction related to a reference origin. The planar polar motion can be extended to 3 dimensional by adding a component of angular elevation to the polar-coordinate system and the coordinates of the new 3D coordinate system is called spherical coordinates.
Component Functions of Spherical Coordinate System
There are three independent coordinates in the spherical coordinate system. The coordinates are radial distance component, azimuthal angular direction component, and polar direction component. In curvilinear motion, when the vector function of motion is resolved into component functions that are radial, transverse and elevation to the reference origin of motion, the orientation of the frame of reference of the object changes during the motion along the path. In other words, the frame of reference of the object is in translative and rotational motions simultaneously along the path of motion. In this case, the position of the object is more convenient to be defined by its spherical coordinates R, θ, and ø instead of rectangular coordinates x, y and z. In fact, the vector sum of the coordinate components for positioning in spherical coordinate system and rectangular coordinate system are the same.
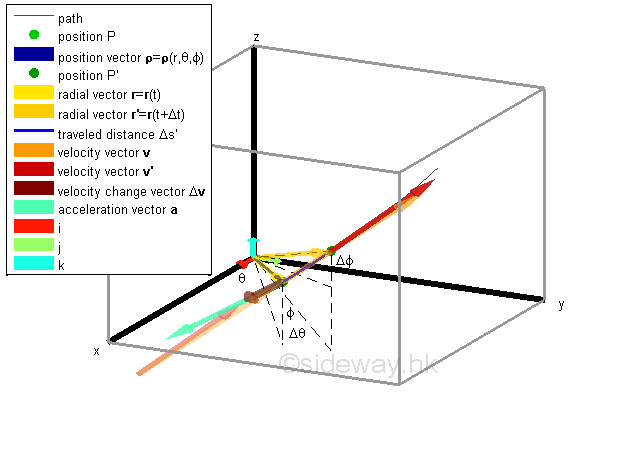
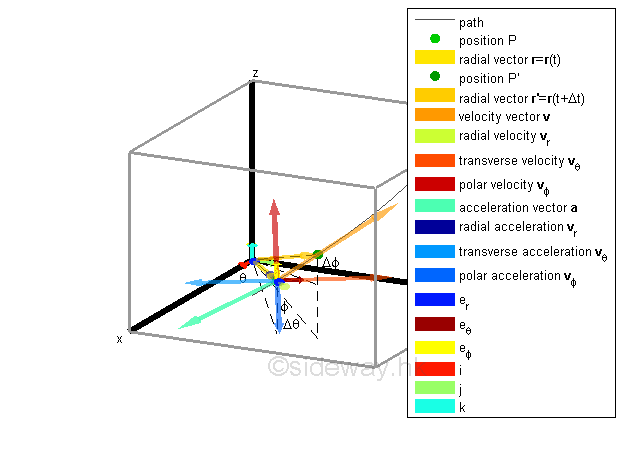
Since the velocity vector of the motion is alway tangent to the path, the vector function of the velocity vector usually have radial, transverse which is parallel to xy-plane as in cylindrical coordinates and normal to the radial, and polar which is normal to radial and transverse velocity components. Similarly the vector function of the acceleration vector also usually have both radial, transverse and polar acceleration components such that the speed of the radial velocity vector can be accelerated by the radial component of the acceleration vector, the directions of the radial velocity vector can be accelerated by the transverse and polar components of the acceleration vector.

Unit Vectors in Spherical Coordinates
Let the unit radial vector of the velocity vector v be er , the unit transverse vector of the velocity vector v be eθ and the unit polar vector of the veloctiy vector v be eø , at position P with reference to the origin O. Unlike the unit vectors i j, and k of the fixed frame of reference, the unit vectors er , eθ and eø are translated and rotated simultaneously along the path of the motion. Sometime the frame of reference used by the observer at the reference origin is more convenient to have the same type of frame of reference. In this case, the position of the object can also be expressed in term of the unit radial vector er , the unit transverse vector eθ and the unit normal vector eθ also. The angle ø and unit polar vector eø are defined same as the unit radial vector by refering to xy instead of zx plane for the unit transverse vector.
Geometrically both the unit radial vector er , the unit transverse vector eθ and the unit polar vector eø can also be expressed in terms of the unit vectors i, j and k of the fixed frame of reference by triangle rule.
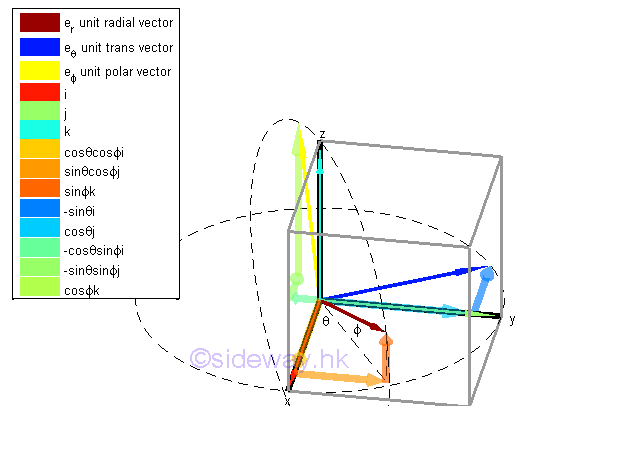
That is .

Unit Vector Derivatives in Spherical Coordinates
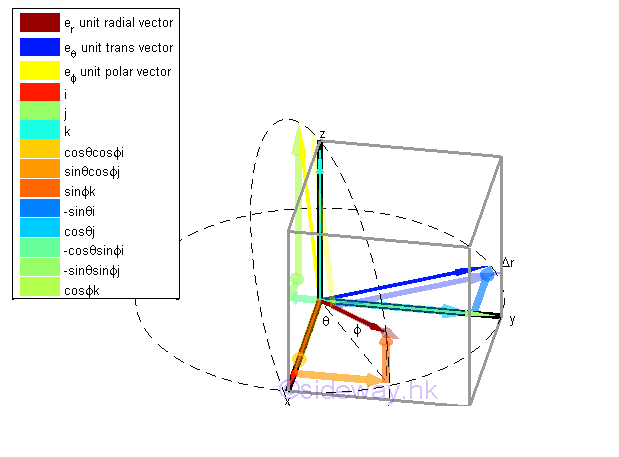
Similar to retangular coordinates, the differential change of the unit vectors in spherical coordinates with respect to the linear displacement along the radial coordinate are always equal to zero, since the magnitudes of unit vectors are always equal to constant and the linear displacement, or a translation does not change the frame of reference. Imply the corresponding differential changes of the unit vectors in spherical coordinates with respect to displacement r is

But for the other two unit vectors related to the angular directional motion, the differential change of the unit vectors in spherical coordinates with respect to the angular directional motion along with the azimuthal and polar angles are usually not equal to zero, the directions of unit vectors may change accordingly although the magnitudes of unit vectors remain constant and the change in angular direction, or a rotation always changes the frame of reference. For the differential change in angle θ.

The corresponding differential changes of the unit vectors in spherical coordinates with respect to angel θ is

For the differential change in angle ø.
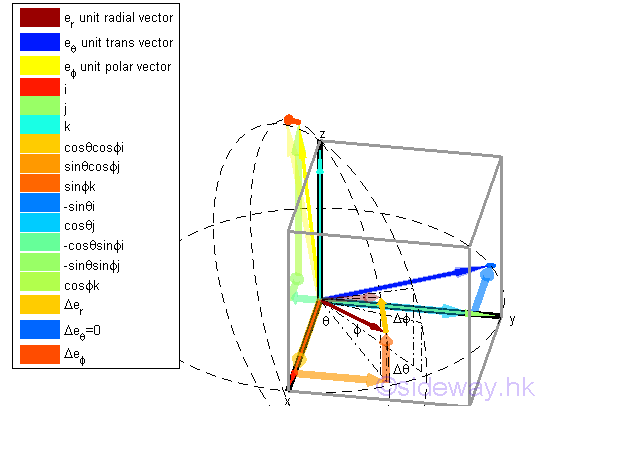
The corresponding differential changes of the unit vectors in spherical coordinates with respect to angle ø is

Position Vector in Spherical Coordinates
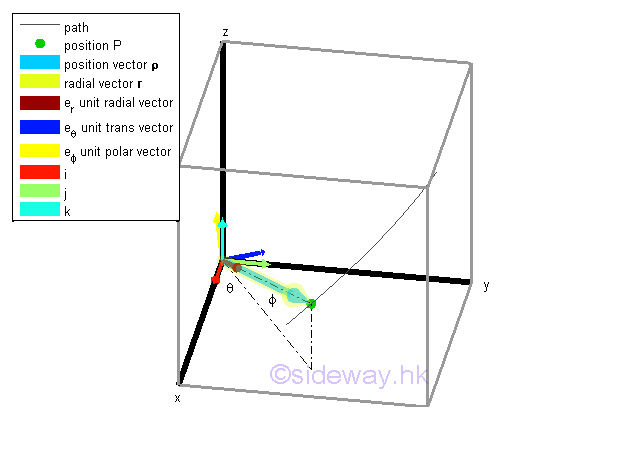
Let the motion of an object at position P is of radial distance r with angles of θ relative to the zx-plane and of ø relative to the xy-plane of the fixed frame of reference at an instant of time t. The position vector ρ of the object can then be expressed in term of the unit radial vector er ,the unit transverse vector eθ and the unit polar vector eø also. Since the radial position vector of the object always aligns with the unit radial vector er, the radial position vector can be expressed in term of the unit radial vector er only, that is rer. Imply

Velocity Vector in Spherical Coordinates
Since the motion of the object can be resolved into radial, transverse and polar motions, the displacement, velocity and aceleration can also be resolved into radial, transverse and polar components accordingly. However as the frame of reference of the radial, transverse and polar components translate and rotate along with the motion, the component of the motion function cannot be obtained from the corresponding component of the change of the related motion function directly as in the fixed frame of reference because the frame of reference rotates along the path of motion besides translating. This is because the method of vector decomposition used in the vector addition with respect to a fixed or translated frame of reference cannot be applied in the case of the addition of vectors with respect to a rotated frame of reference. In other words, the velocity components cannot be obtained from the position vector components directly.
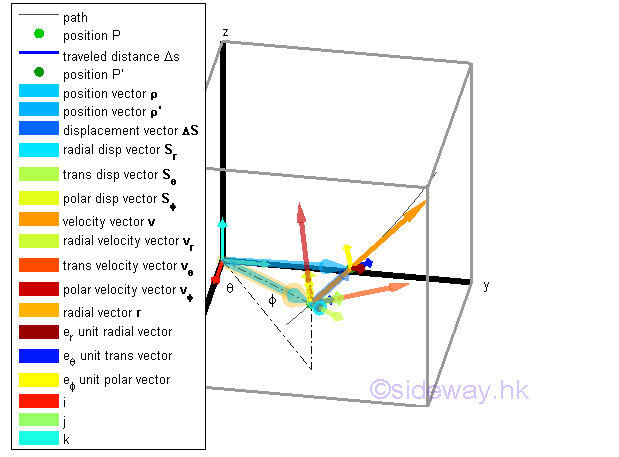
That is, the displacement of the object with radial position vector rer is equal to a displacement vector function with radial, transverse and polar components instead of only radial component because the frame of reference of the position vector at the end of the motion at position P' is rotated with respect to the frame of reference of the displacement vector although both position vector and displacement vector have the same frame of reference at the beginning of the motion at position P. However, as the frame of reference of a velocity vector is always same as the displacement vector, the component functions of the velocity vector can be obtained directly from the corresponding components of the displacement vector. While the acceleration vector of the motion is similar to the case of displacement vector.

Or by differentiation, imply
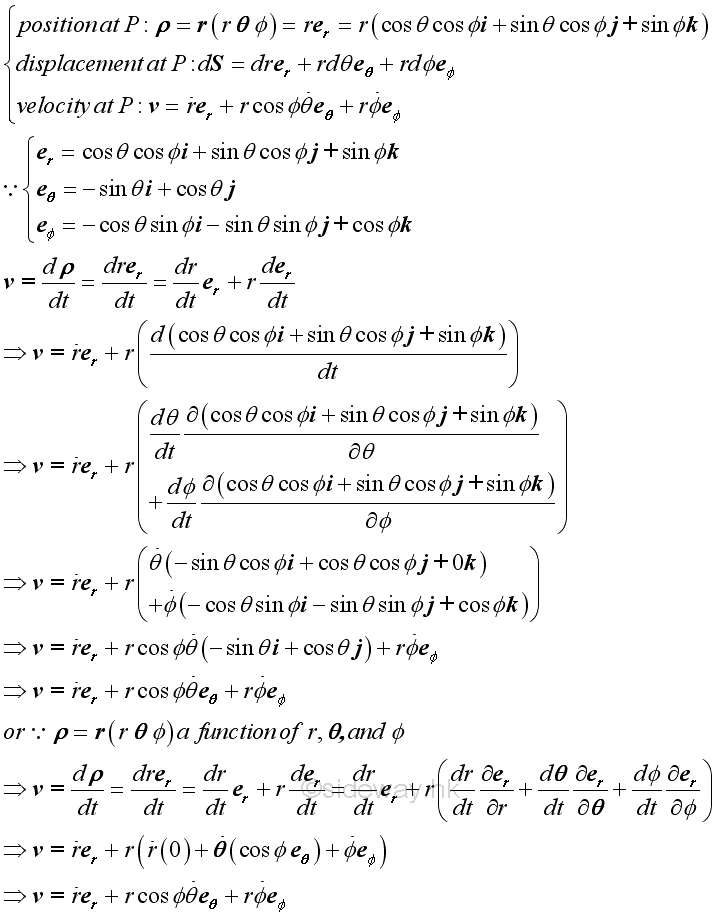
Acceleration Vector in Spherical Coordinates
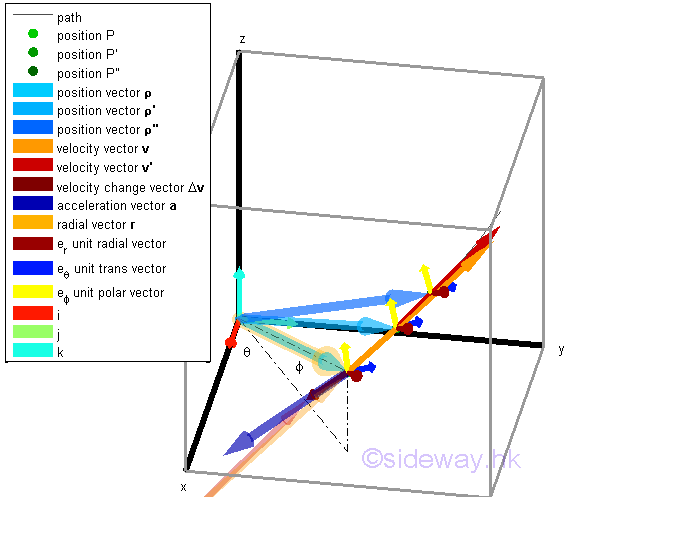
By definition, the velocity vector function of a motion is equal to the rate of change of the displacement vector function, therefore the velocity vector function of a motion can be derived from the displacement vector function directly. And by definition, the acceleration vector function of a motion is equal to the rate of change of the veclocity vector function, therefore the velocity vector function of a motion can be derived from the displacement vector function using differentiation also. Imply

or
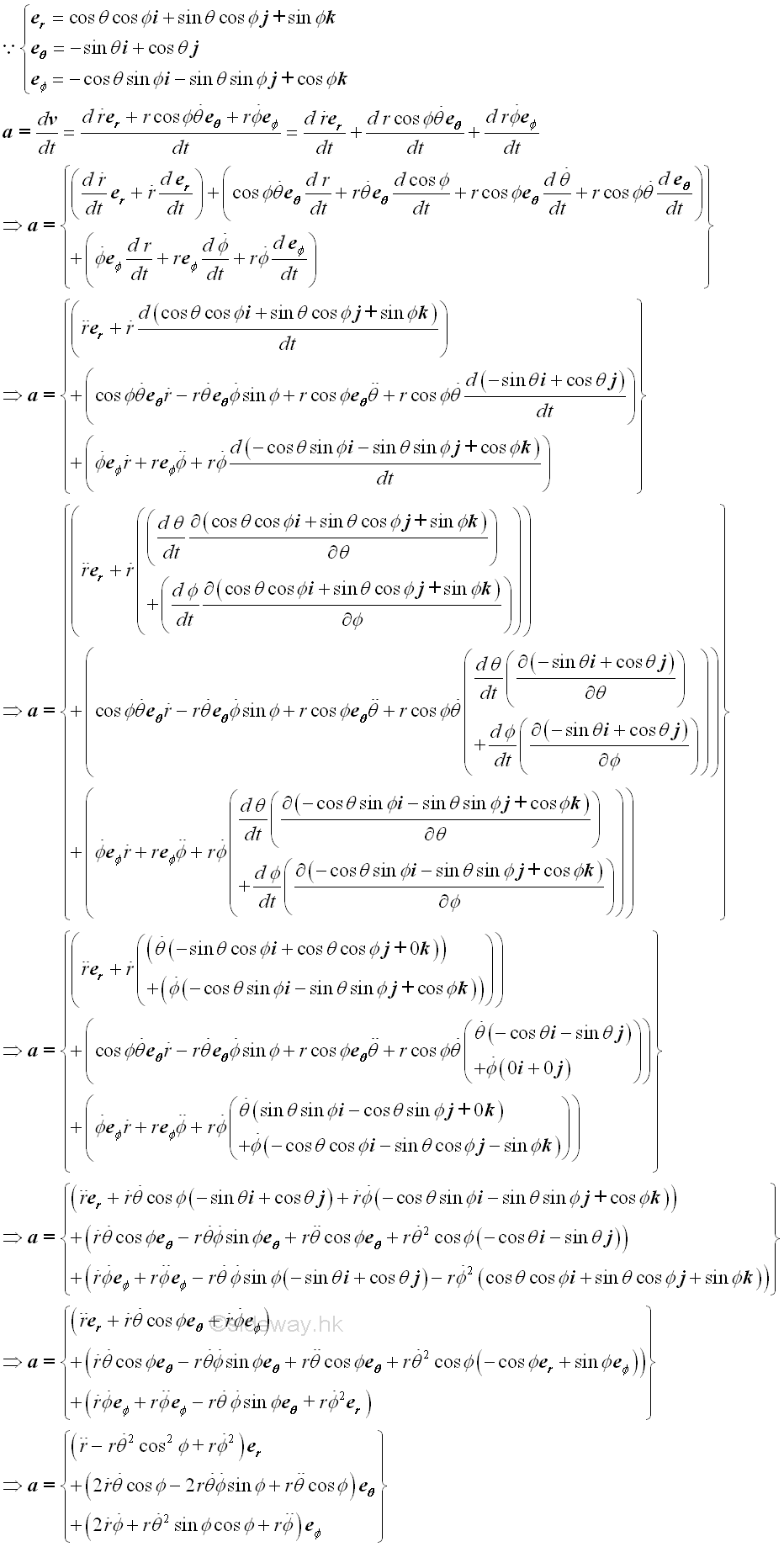
Motion Functions in Spherical Coordinates
Therefore the motion functions of an object in spherical coordinates are.
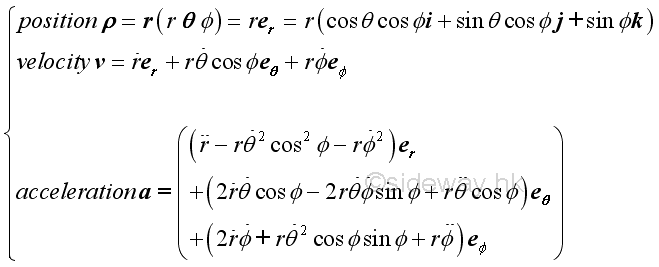
©sideway
ID: 140700003 Last Updated: 7/18/2014 Revision: 1 Ref:
References
- I.C. Jong; B.G. rogers, 1991, Engineering Mechanics: Statics and Dynamics
- F.P. Beer; E.R. Johnston,Jr.; E.R. Eisenberg, 2004, Vector Mechanics for Engineers: Statics
Latest Updated Links
- Pentium(last updated On 7/3/2025)
- Intel CPU(last updated On 7/2/2025)
- Intel CPU History(last updated On 7/1/2025)
- FreeGLUT Windows Function(last updated On 1/27/2025)
- FreeGLUT Initialization Function(last updated On 1/26/2025)
- FreeGLUT(last updated On 1/25/2025)
- GLUT(last updated On 1/24/2025)
- OpenGL(last updated On 1/23/2025)
- XPower UC140 4-Port 140W PD3.1 GaN Travel Charger(last updated On 1/22/2025)
- XPower DX6 6 In 1 (2x3) 60W PD3.0 Sync & Charge Cable(last updated On 1/21/2025)
- XPower MF240 1.2M Magnetic Absorption 4 in 1 (2x2) Zinc Alloy 240W PD Sync & Charge Cable(last updated On 1/20/2025)

 Nu Html Checker
Nu Html Checker  53
53  na
na  na
na
Home 5
Business
Management
HBR 3
Information
Recreation
Hobbies 8
Culture
Chinese 1097
English 339
Travel 18
Reference 79
Computer
Hardware 257
Software
Application 213
Digitization 37
Latex 52
Manim 205
KB 1
Numeric 19
Programming
Web 289
Unicode 504
HTML 66
CSS 65
SVG 46
ASP.NET 270
OS 431
DeskTop 7
Python 72
Knowledge
Mathematics
Formulas 8
Set 1
Logic 1
Algebra 84
Number Theory 206
Trigonometry 31
Geometry 34
Calculus 67
Engineering
Tables 8
Mechanical
Rigid Bodies
Statics 92
Dynamics 37
Fluid 5
Control
Acoustics 19
Natural Sciences
Matter 1
Electric 27
Biology 1
