Content
Instantaneous Rate of Change
Linear Motion
Linear Motion Problem
Instantaneous Rate of Change
Rate of change is the average change of function y=ƒ(x) between two instants, x and x+Δx. The derivative is the instantaneous rate of change by applying limit Δx to zero.
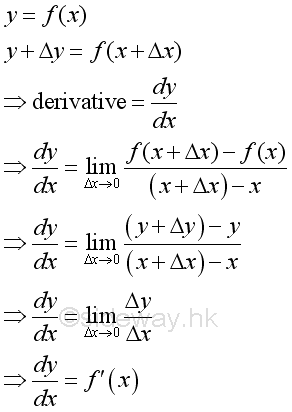
Linear Motion
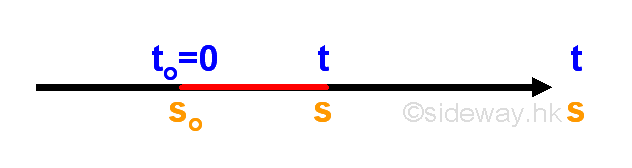
For a linear motion with uniform speed v, the relationship between the travelled distance s, initial distance so, speed v and time t can be expressed as a function:

Instantaneous velocity can be defined as the instantaneous rate of change of the travelled distance with respect to the time of travelling. The derivative of the function s with respect to time t is.
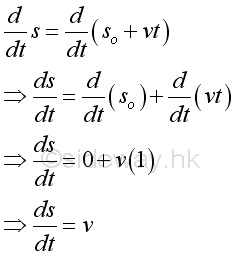
For a linear motion with constant acceleration a, the relationship between the final speed v, initial speed vo, acceleration a and time t can be expressed as a function:
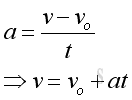
Instantaneous acceleration can be defined as the instantaneous rate of change of the travelling velocity with respect to the time of travelling. The derivative of the function v with respect to time t is.
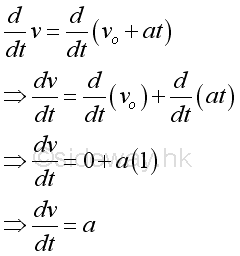
Since s is a function of time f(t), the derivative v is equal to the first derivative of function s with respect to t.
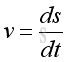
The derivative a is equal to the derivative of function v with respect to time t. But v is also a derivative of function s with respect to time t, the derivative is
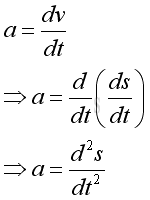
Therefore the derivative a is also equal to the second derivative of function s with respect to t.
Linear Motion Problem
For an object with a linear motion and the motion can be described by a distance function s with respect to time t.
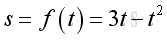
Graphically
The information directly from the function is the travelled distance from the original location.
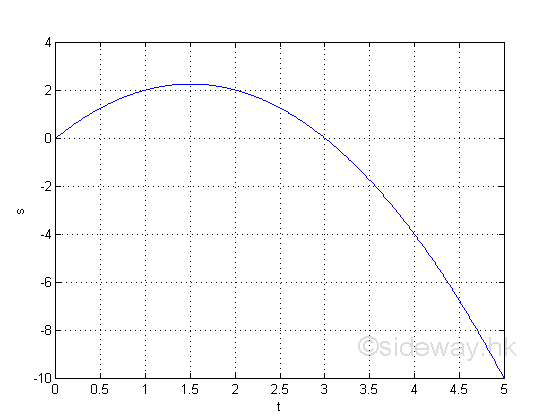
e.g. at t=1, the distance from the original location is 2,
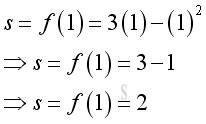
e.g. at t=2, the distance from the original location is 2

e.g. at t=4, the distance from the original location is -4, the negative sign means the distance is measured from the opposite side of the original location relative to the original default measurement.
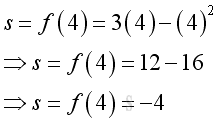
The first derivative can be used to determine the instantaneous verlocity of the object motion. imply
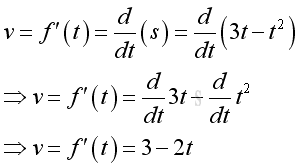
Graphically
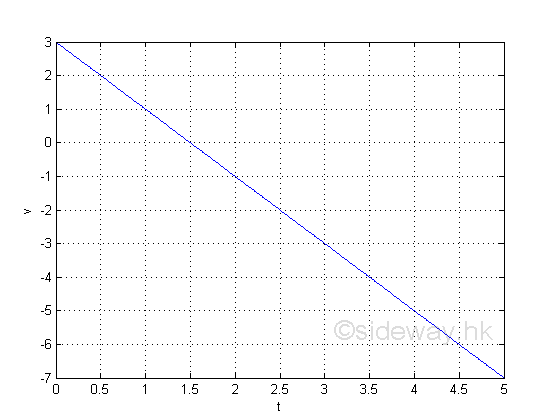
The information directly from the derivative is the instantaneous velocity.
e.g. at t=1, the instantaneous velocity is 1
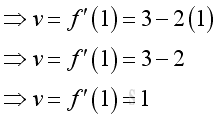
e.g. at t=2, the instantaneous velocity is -1, the negative sign means the direction of motion is opposite to the originial direction of the default motion.
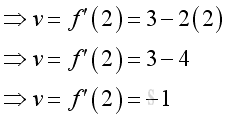
e.g. In stationary state condition, the instantaneous velocity of the object is eqaul to zero. Imply
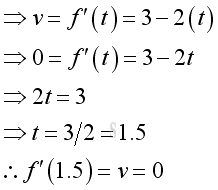
Therefore at t=1.5, the object remains stationary at its location with a zero instantaneous velocity. Imply the location is
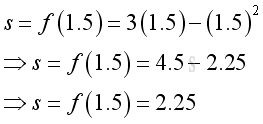
The second derivative can be used to determine the instantaneous acceleration of the object motion. imply
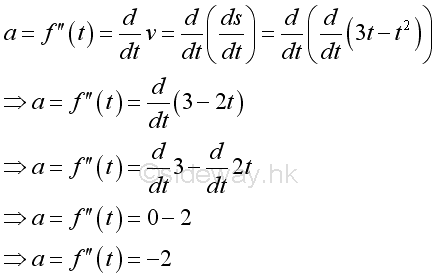
The accelation is equal to -2, a negative acceleration. The value is a negative constant which imply it is a constant deceleration. And therefore the instantaneous velocity is decreasing with respect to time. Graphically, the acceleration is
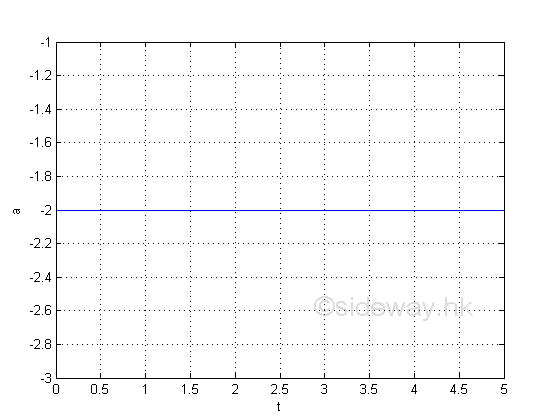
©sideway
ID: 110900014 Last Updated: 9/22/2001 Revision: 1 Ref:
References
- S. James, 1999, Calculus
- B. Joseph, 1978, University Mathematics: A Textbook for Students of Science & Engineering
Latest Updated Links
- Pentium(last updated On 7/3/2025)
- Intel CPU(last updated On 7/2/2025)
- Intel CPU History(last updated On 7/1/2025)
- FreeGLUT Windows Function(last updated On 1/27/2025)
- FreeGLUT Initialization Function(last updated On 1/26/2025)
- FreeGLUT(last updated On 1/25/2025)
- GLUT(last updated On 1/24/2025)
- OpenGL(last updated On 1/23/2025)
- XPower UC140 4-Port 140W PD3.1 GaN Travel Charger(last updated On 1/22/2025)
- XPower DX6 6 In 1 (2x3) 60W PD3.0 Sync & Charge Cable(last updated On 1/21/2025)
- XPower MF240 1.2M Magnetic Absorption 4 in 1 (2x2) Zinc Alloy 240W PD Sync & Charge Cable(last updated On 1/20/2025)

 Nu Html Checker
Nu Html Checker  53
53  na
na  na
na
Home 5
Business
Management
HBR 3
Information
Recreation
Hobbies 8
Culture
Chinese 1097
English 339
Travel 18
Reference 79
Computer
Hardware 257
Software
Application 213
Digitization 37
Latex 52
Manim 205
KB 1
Numeric 19
Programming
Web 289
Unicode 504
HTML 66
CSS 65
SVG 46
ASP.NET 270
OS 431
DeskTop 7
Python 72
Knowledge
Mathematics
Formulas 8
Set 1
Logic 1
Algebra 84
Number Theory 206
Trigonometry 31
Geometry 34
Calculus 67
Engineering
Tables 8
Mechanical
Rigid Bodies
Statics 92
Dynamics 37
Fluid 5
Control
Acoustics 19
Natural Sciences
Matter 1
Electric 27
Biology 1
