Content
Trigonometry
Radius of Incircle
Radius of Excircle
Trigonometry
Trigonometric functions are related with the properties of triangles. Some laws and formulas are also derived to tackle the problems related to triangles, not just right-angled triangles.
Radius of Incircle

Consider a circle incscrbed in a triangle ΔABC with centre O and radius r, the tangent function of one half of an angle of a triangle is equal to the ratio of the radius r over the sum of two sides adjacent to the angle. Since the incircle for the three different types of angled triangles can be constructed in similar way, the proofs for acute angled triangle with all the internal angles are less than π/2, the right angled triangle with one of the internal angles is equal to π/2, and the obtuse angled triangle with one of the internal angles is greater than π/2 but less than π are the same. For a circle incscrbed in a triangle ΔABC with centre O and radius r, three triangles, ΔAOB, ΔBOC, and ΔCOB, can be constructed by joining the vertices, A, B, and C to the intersection of the three angle bisectors of the triangle, O with the same altitude of r. Imply

Consider triangle ΔCOB, imply

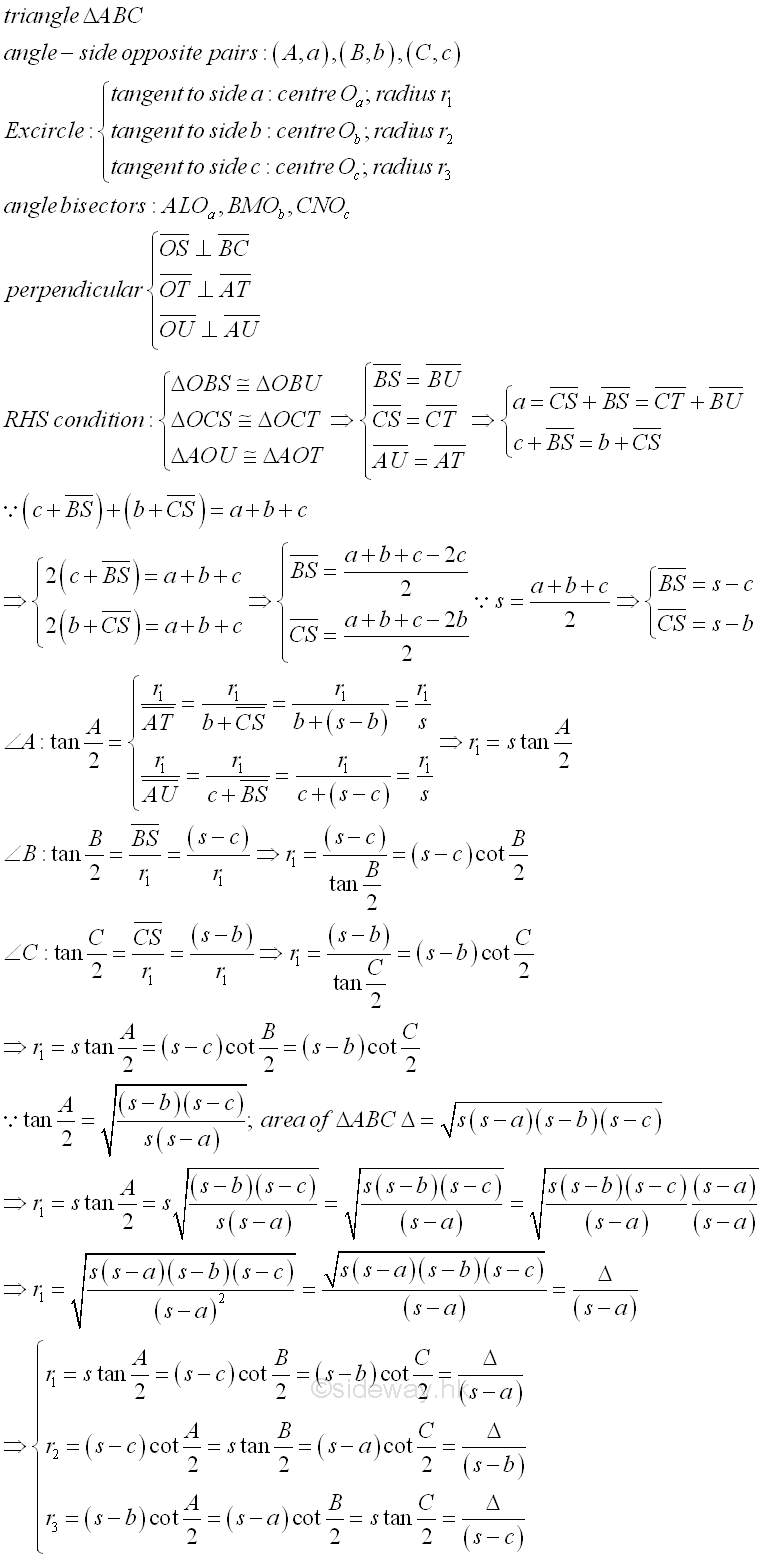
Radius of Excircle

Unlike incirlce of a triangle, an excircle is constructued outside the triangle with one side and two extended lines of the triangle are tangent to the circle. The tangent function of one half of an angle of a triangle is equal to the ratio of the radius r of the circle tangent to the side opposite over the sum of three sides. Since the excircle for the three different types of angled triangles can be constructed in similar way, the proofs for acute angled triangle with all the internal angles are less than π/2, the right angled triangle with one of the internal angles is equal to π/2, and the obtuse angled triangle with one of the internal angles is greater than π/2 but less than π are the same. But the excircle tangent to each side of the triangle may not be of the same radius, the three excircles are therefore of centres Oa, Ob, and Oc and with radius r1, r2, and r3. Besides each excircle can be expressed in terms of the three angles of the triangle separately. Imply
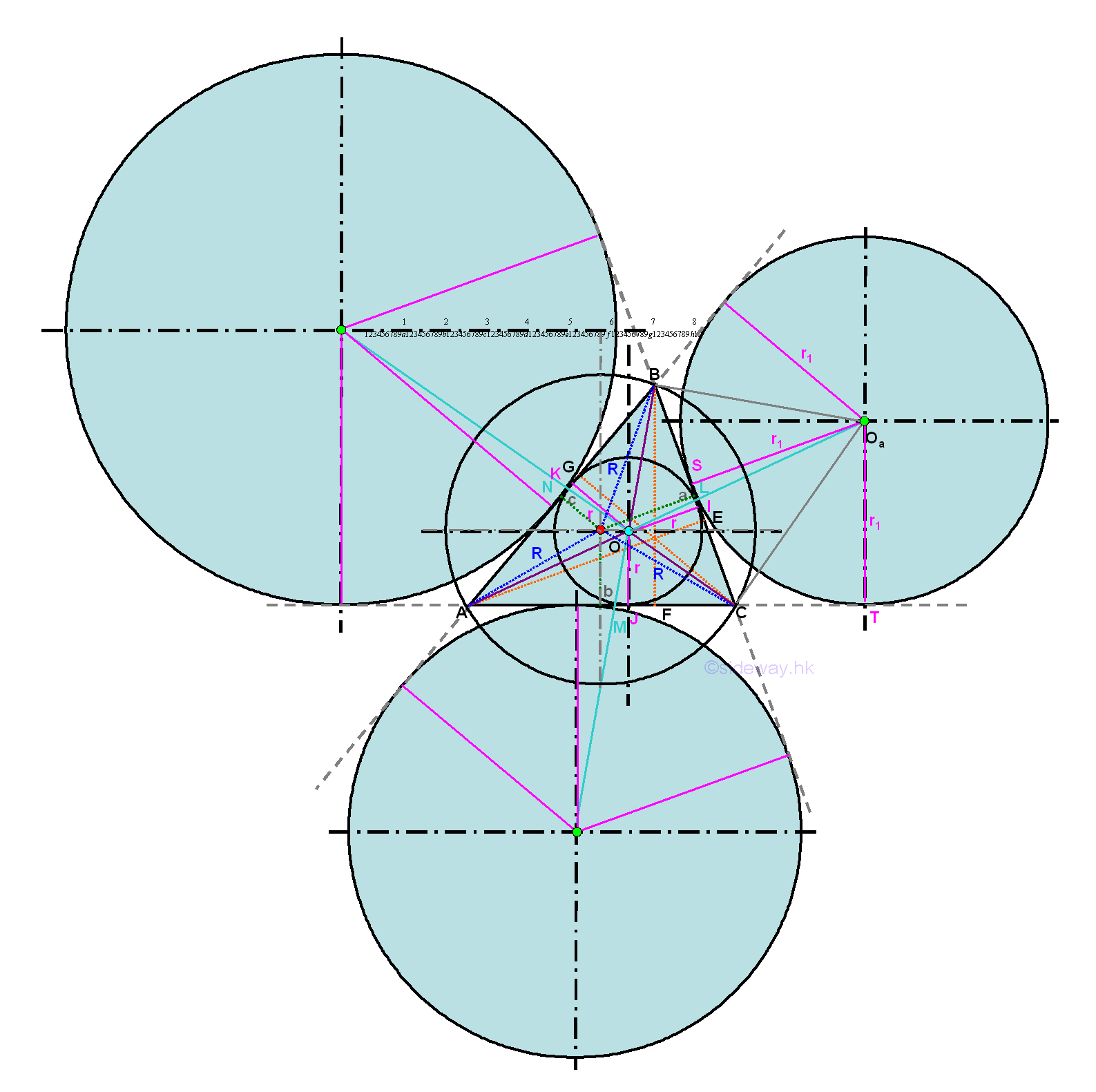
Consider excircle tangent side b, imply
©sideway
ID: 130600034 Last Updated: 7/1/2013 Revision: 1 Ref:
References
- B. Joseph, 1978, University Mathematics: A Textbook for Students of Science & Engineering
- Ayres, F. JR, Moyer, R.E., 1999, Schaum's Outlines: Trigonometry
- Hopkings, W., 1833, Elements of Trigonometry
Latest Updated Links
- Pentium(last updated On 7/3/2025)
- Intel CPU(last updated On 7/2/2025)
- Intel CPU History(last updated On 7/1/2025)
- FreeGLUT Windows Function(last updated On 1/27/2025)
- FreeGLUT Initialization Function(last updated On 1/26/2025)
- FreeGLUT(last updated On 1/25/2025)
- GLUT(last updated On 1/24/2025)
- OpenGL(last updated On 1/23/2025)
- XPower UC140 4-Port 140W PD3.1 GaN Travel Charger(last updated On 1/22/2025)
- XPower DX6 6 In 1 (2x3) 60W PD3.0 Sync & Charge Cable(last updated On 1/21/2025)
- XPower MF240 1.2M Magnetic Absorption 4 in 1 (2x2) Zinc Alloy 240W PD Sync & Charge Cable(last updated On 1/20/2025)

 Nu Html Checker
Nu Html Checker  53
53  na
na  na
na
Home 5
Business
Management
HBR 3
Information
Recreation
Hobbies 8
Culture
Chinese 1097
English 339
Travel 18
Reference 79
Computer
Hardware 257
Software
Application 213
Digitization 37
Latex 52
Manim 205
KB 1
Numeric 19
Programming
Web 289
Unicode 504
HTML 66
CSS 65
SVG 46
ASP.NET 270
OS 431
DeskTop 7
Python 72
Knowledge
Mathematics
Formulas 8
Set 1
Logic 1
Algebra 84
Number Theory 206
Trigonometry 31
Geometry 34
Calculus 67
Engineering
Tables 8
Mechanical
Rigid Bodies
Statics 92
Dynamics 37
Fluid 5
Control
Acoustics 19
Natural Sciences
Matter 1
Electric 27
Biology 1
