Content
Successive Differentiation
nᵗʰ
Derivative of
Function
Successive Differentiation
In general, the differentiation of a function can be differentiated succesively to obtain Higher Order Derivatives. Let function y=f(x) be a function of x. Then the result of differentiating y with respect to x is defined as the derivative or the first derivative of y with respect to x and is denoted as
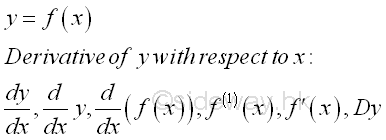
Then the result of differentiating ƒ′(x) with respect to x is defined the second differential derivative of y with respect to x and is denoted as
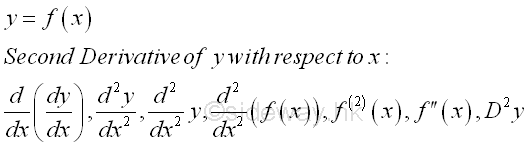
Similarly, the result of differentiating ƒ″(x) with respect to x is defined the third differential derivative of y with respect to x and is denoted as

By continuing the differentiation process, the result of differentiating ƒ(x) n times with respect to x successively is defined the nth differential derivative of y with respect to x and is denoted as
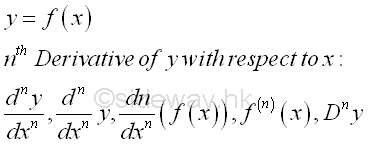
The second, third, fourth, ..., nth derivatives are called higher order derivatives.
nᵗʰ Derivative of Function
-
nth Derivative of General Polynomial Function
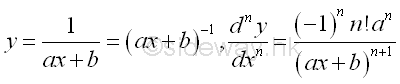
Proof:
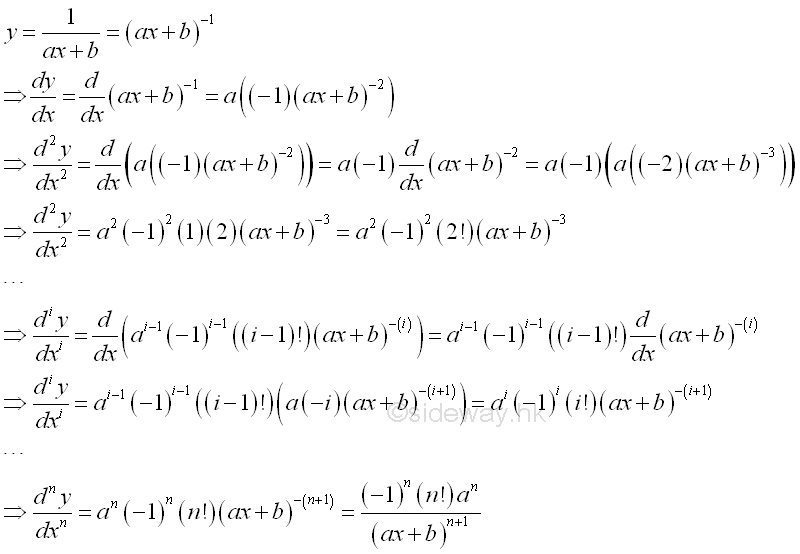
-
nth Derivative of General Natural Exponential Function
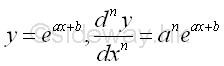
Proof:
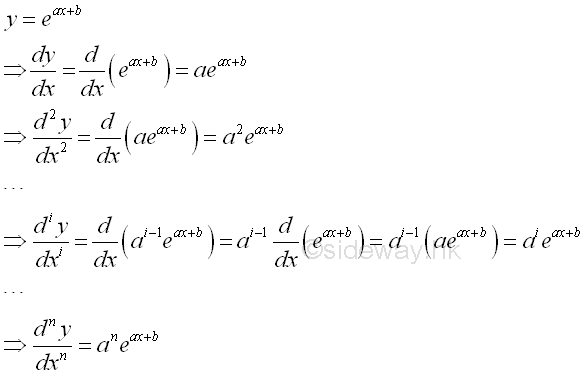
-
nth Derivative of General Exponential Function
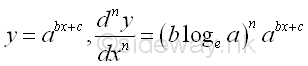
Proof:
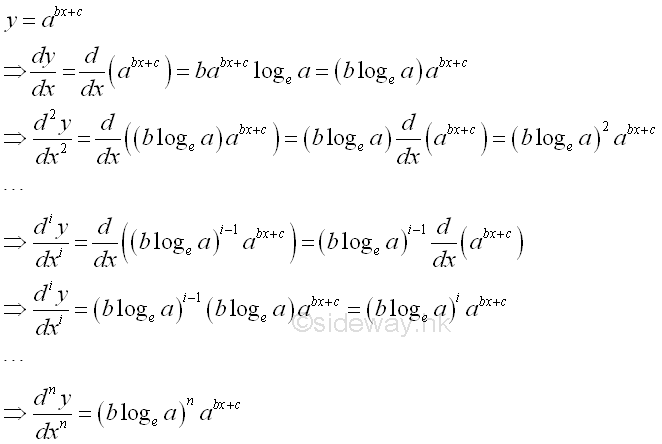
-
nth Derivative of General Natural Logarithmic Function
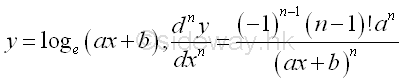
Proof:

-
nth Derivative of General Sine Function

Proof:
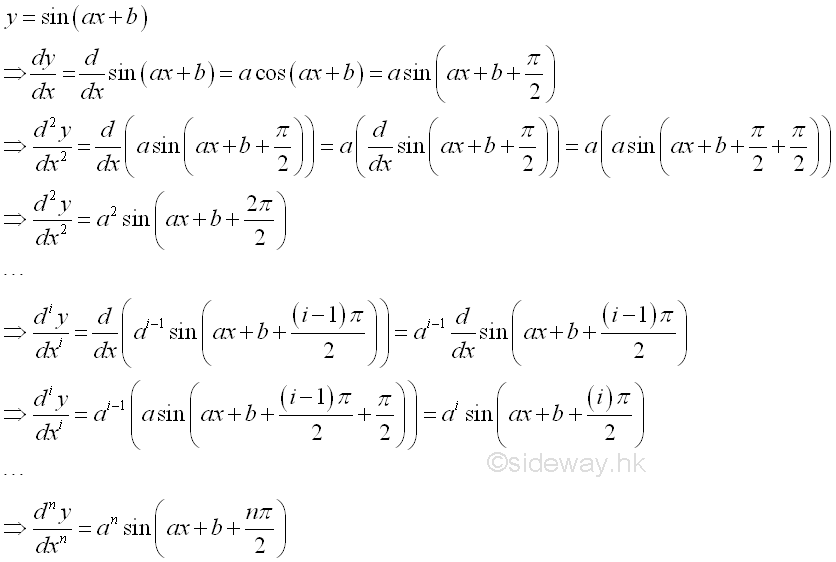
-
nth Derivative of General Cosine Function
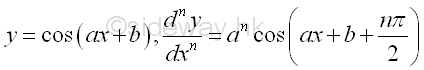
Proof:
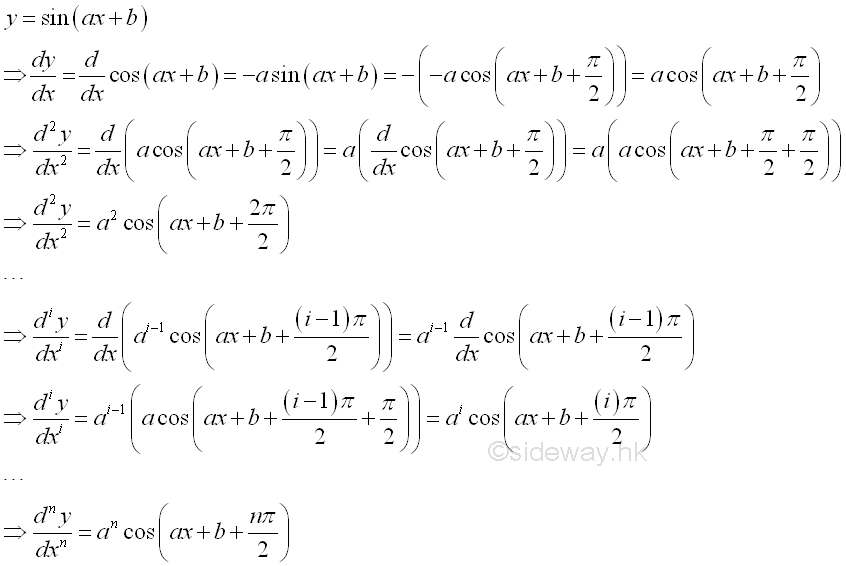
-
nth Derivative of Product of General Functions
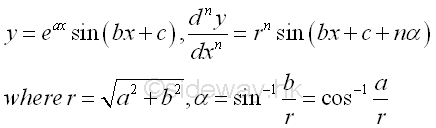
Proof:

-
nth Derivative of Product of General Functions
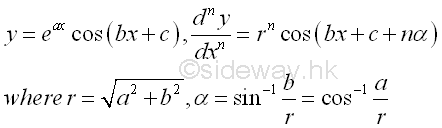
Proof:

-
nth Derivative of Product of Functions (Leibnitz's Theorem)
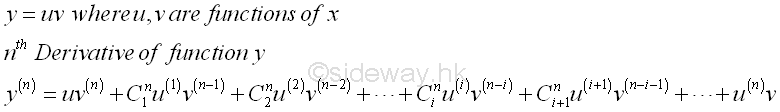
Proof:

©sideway
ID: 130700029 Last Updated: 7/15/2013 Revision: 0 Ref:
References
- S. James, 1999, Calculus
- B. Joseph, 1978, University Mathematics: A Textbook for Students of Science & Engineering
Latest Updated Links
- Pentium(last updated On 7/3/2025)
- Intel CPU(last updated On 7/2/2025)
- Intel CPU History(last updated On 7/1/2025)
- FreeGLUT Windows Function(last updated On 1/27/2025)
- FreeGLUT Initialization Function(last updated On 1/26/2025)
- FreeGLUT(last updated On 1/25/2025)
- GLUT(last updated On 1/24/2025)
- OpenGL(last updated On 1/23/2025)
- XPower UC140 4-Port 140W PD3.1 GaN Travel Charger(last updated On 1/22/2025)
- XPower DX6 6 In 1 (2x3) 60W PD3.0 Sync & Charge Cable(last updated On 1/21/2025)
- XPower MF240 1.2M Magnetic Absorption 4 in 1 (2x2) Zinc Alloy 240W PD Sync & Charge Cable(last updated On 1/20/2025)

 Nu Html Checker
Nu Html Checker  53
53  na
na  na
na
Home 5
Business
Management
HBR 3
Information
Recreation
Hobbies 8
Culture
Chinese 1097
English 339
Travel 18
Reference 79
Computer
Hardware 257
Software
Application 213
Digitization 37
Latex 52
Manim 205
KB 1
Numeric 19
Programming
Web 289
Unicode 504
HTML 66
CSS 65
SVG 46
ASP.NET 270
OS 431
DeskTop 7
Python 72
Knowledge
Mathematics
Formulas 8
Set 1
Logic 1
Algebra 84
Number Theory 206
Trigonometry 31
Geometry 34
Calculus 67
Engineering
Tables 8
Mechanical
Rigid Bodies
Statics 92
Dynamics 37
Fluid 5
Control
Acoustics 19
Natural Sciences
Matter 1
Electric 27
Biology 1
