Content
Internal Forces
in Beams
Shear and Bending Moment
Diagrams
Internal Forces in a Simply Supported Beam with Distributed Load
Shear Diagramar Diagram
Bending Moment Diagram
Internal Forces in a Simply Supported Beam
with Concentrated Load and Concentrated Moment
Shear Diagram
Bending Moment Diagram
Internal Forces in a Simply Supported Beam
with a Concentrated Couple
Shear Diagram
Bending Moment Diagram
Internal Forces in Beams
The shearing forces and bending moments in a statically determinate beam can be determined by the equilibrium equations. Using the standard convention, the distribution of internal forces in a beam can be represented graphically by plotting the values of shear or bending moment against the distance from one end of the beam. Besides concentrated applied loads and moments, the applied force can also be distributed applied loads and moments, and concentrated couples.
Shear and Bending Moment Diagrams
Internal Forces in a Simply Supported Beam with Distributed Load
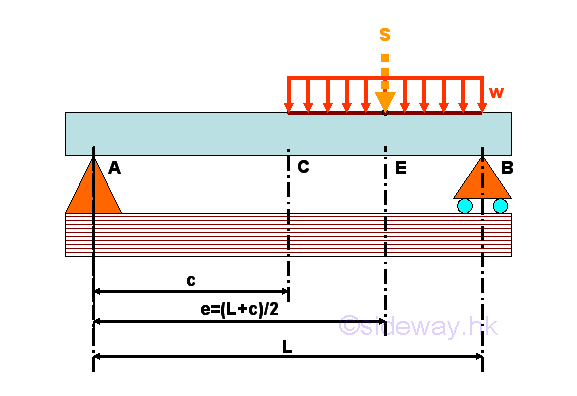
For example, consider a simply supported beam with a distributed load of w per unit length over length CB of the beam. The equivalent force for the distributed load can be represented by a force S acting at the middle point E of length CB on the beam, i.e. e=(L+c)/2. The free-body diagram of the entire beam is
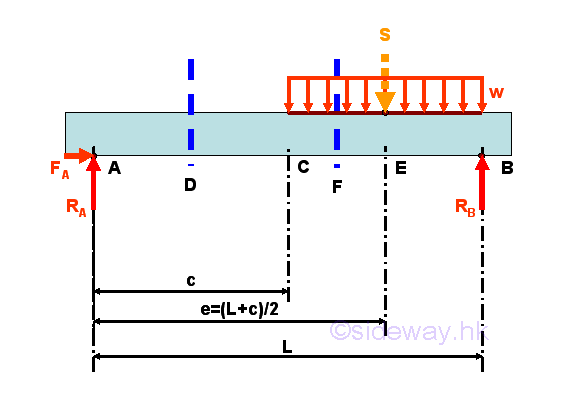
The reactions at the hinged and roller supports can be determined by the equilibrium equations.
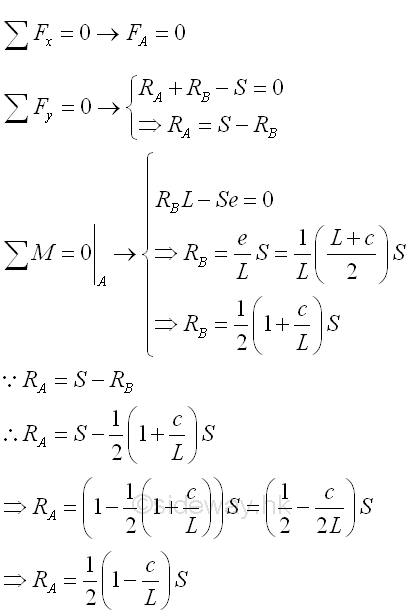
Internal forces can be determined by dividing the beam into two separated free body. Selecting a point D between A and C, i.e. A<D<C
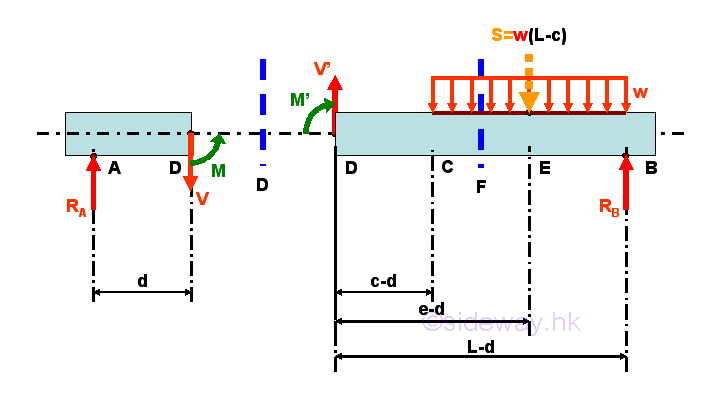
Consider the member section AD of length d, the internal forces at point D are
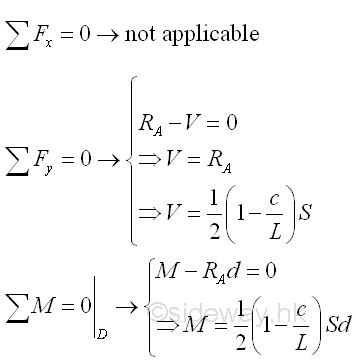
Point D is a random point between A and C. The shear force V is a constant and is equal to (1-c/L)S/2 between point A and point D. And bending moment M is a linear function of point D. Therefore bending moment M=0 at point A and bending moment M=(1-c/L)Sc/2 at point C.
Selecting another point F between C and B, i.e. C<F<B
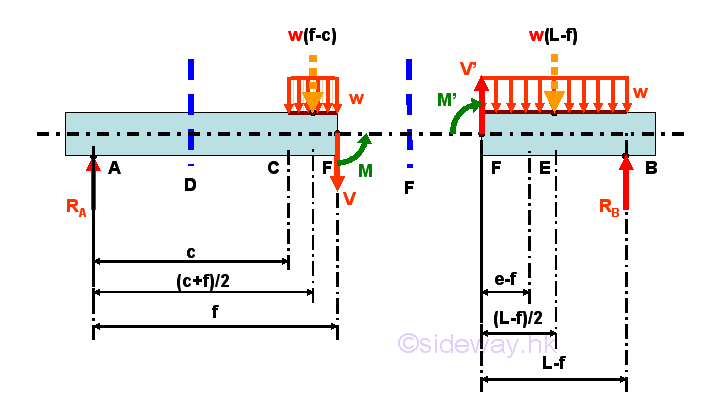
Consider the member section AF of length L-F, the internal forces at point F are
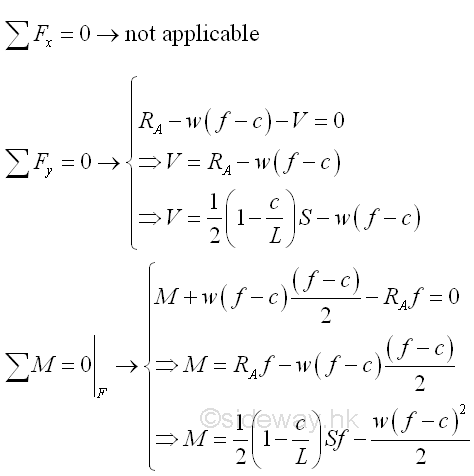
Point F is a random point between C and B. The shear force V is a linear function of point D.The shear force V=(1-c/L)S/2 at point C and the shear force V=(1-c/L)S/2-w(L-c)=-(1+c/L)S/2 at point B. Because of the moment due to the distributed load, the bending moment M is also a second degree function of point D. Therefore bending moment M=(1-c/L)Sc/2 at point C and bending moment M=(1-c/L)SL/2-w(L-c)^2/2=((L-c)-(L-c))S/2=0 at point B. However, the bending moment M is a curve of degree n between C and B, more data is needed to plot the bending moment curve between C and B. The curve of bending moment M between C and B can be rewritten as
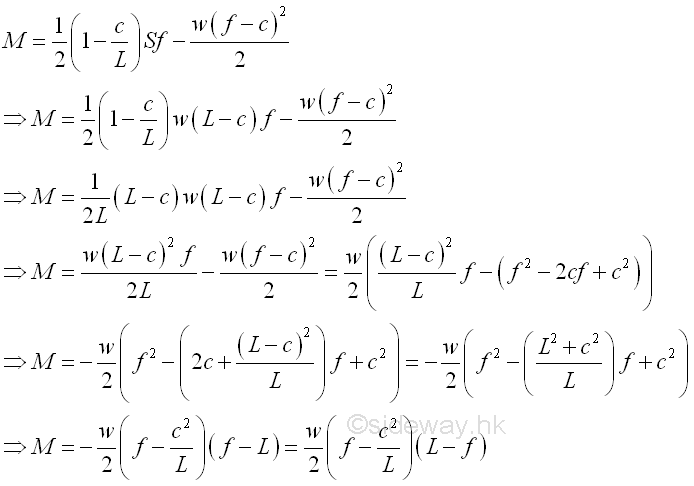
Therefore the bending moment M between C and B is always greater or equal to zero. The maximum bending moment M between C and B is
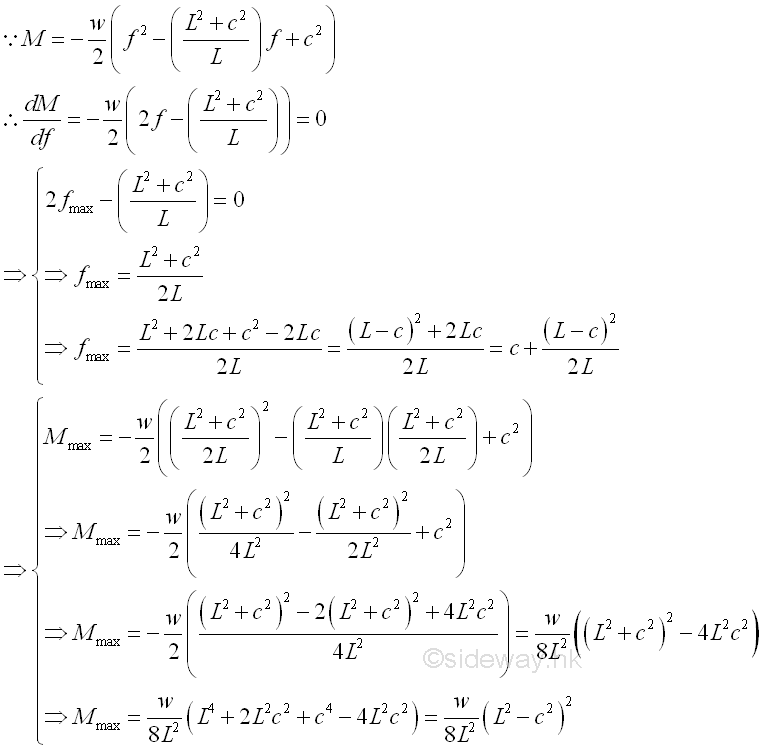
And the bending moments M between C and B at typical points are
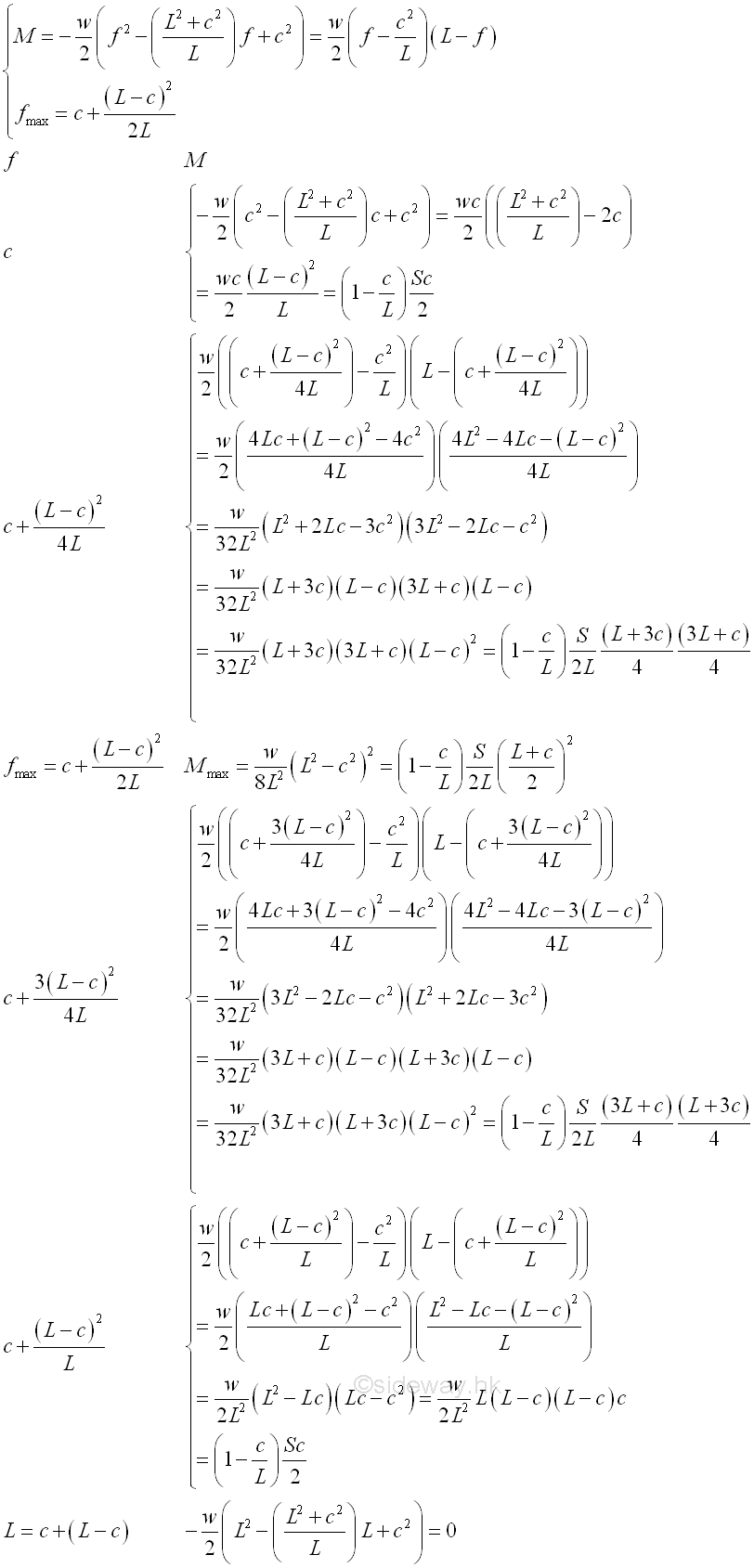
similarly when the shear is equal to zero, the position of point F is

Shear Diagramar Diagram

Bending Moment Diagram

Internal Forces in a Simply Supported Beam with Concentrated Load and Concentrated Moment
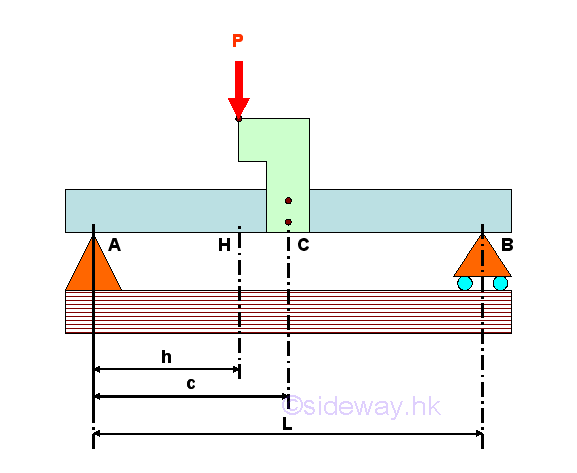
For example, consider a simply supported beam with a concentrated load and a concentrate moment by applying one applied force on the L shape plate which is fixed on around the middle of the beam. Since the applied force P is not directly acting on the beam body, the applied force P applied on the L shape will generated a concentrated force P and a concentrated moment MC at the mounting point of the L shape plate on the beam body. Through system of force transformation, the generated concentrated moment due to the applied force P at point C is
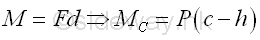
The free-body diagram of the entire beam is

The reactions at the hinged and roller supports can be determined by the equilibrium equations.
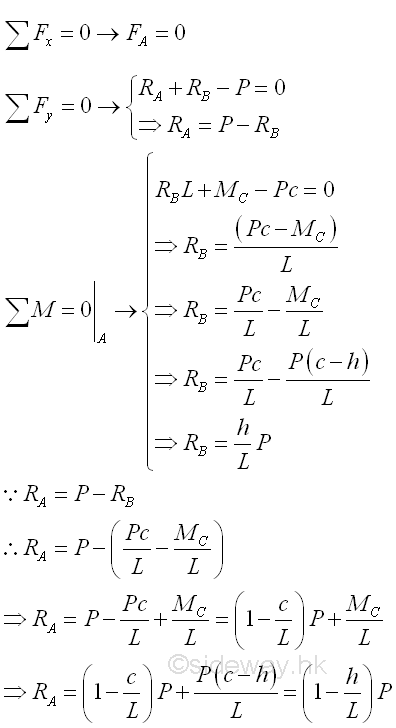
Internal forces can be determined by dividing the beam into two separated free body. Selecting a point D between A and C, i.e. A<D<C

Consider the member section AD of length d, the internal forces at point D are
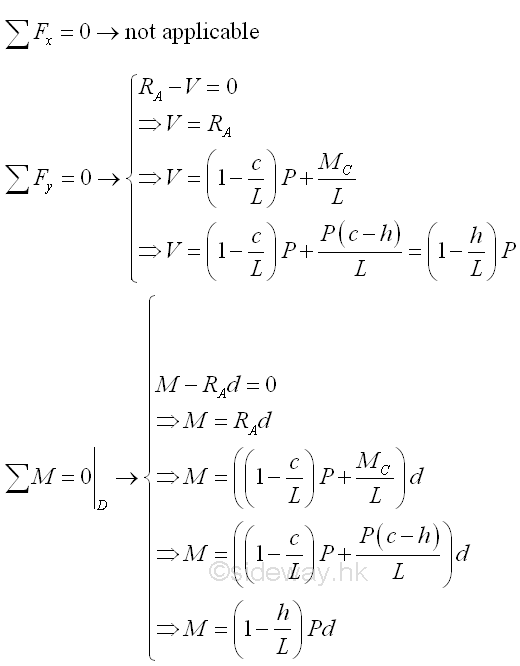
Point D is a random point between A and C. The shear force V is a constant and is equal to (1-h/L)P between point A and point C. And bending moment M is a linear function of point D. Therefore bending moment M=0 at point A and bending moment M=(1-h/L)Pc at point C.
Selecting another point E between C and B, i.e. C<E<B
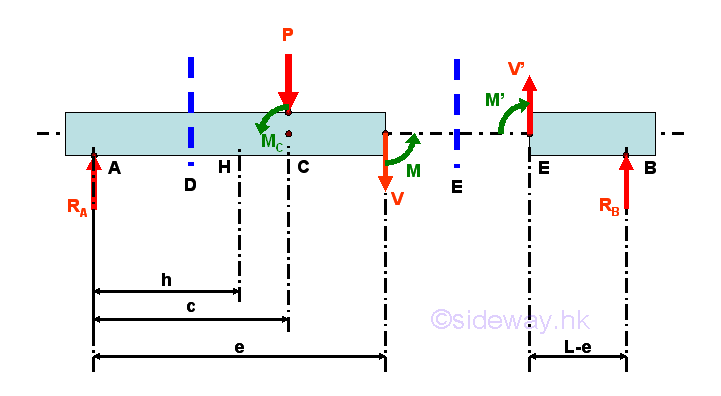
Consider the member section EB of length L-e, the internal forces at point E are

Point E is a random point between C and B. The shear force V is a constant and is equal to -(h/L)P between point C and point B. And bending moment M is a linear function of point E. Therefore bending moment M=(1-c/L)Ph at point C and bending moment M=0 at point B.
Shear Diagram
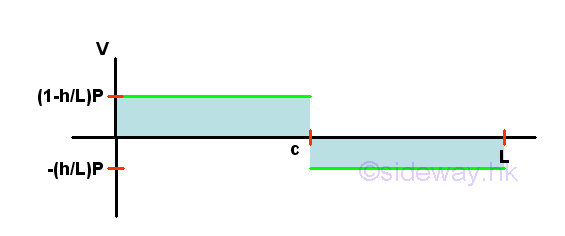
Bending Moment Diagram
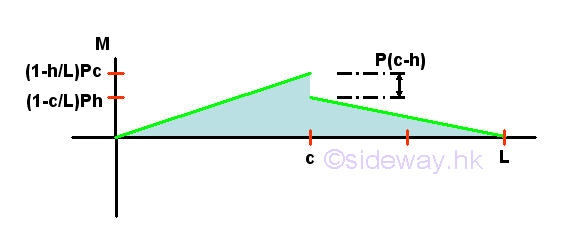
Internal Forces in a Simply Supported Beam with a Concentrated Couple
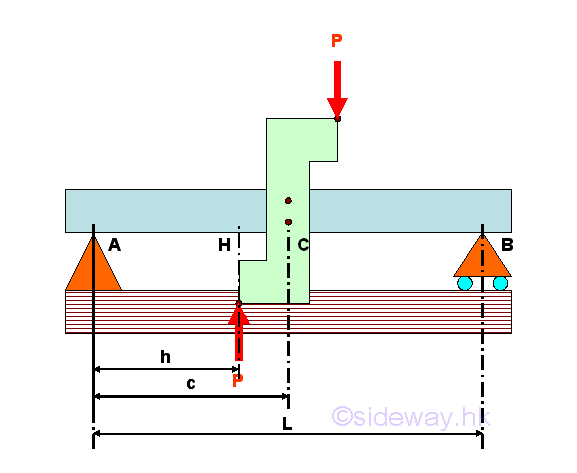
For example, consider a simply supported beam with a concentrated couple by applying a couple, a pair of applied forces P on the S shape plate which is fixed on around the middle of the beam. Since the pair of applied forces P is not directly acting on the beam body, the pair of applied forces P applied on the L shape will generated a concentrated couple at the mounting point of the S shape plate on the beam body only. And the resultant force due the pair of the applied forces is equal to zero. Through system of force transformation, the generated concentrated moment due to the pair of applied force P at point C is
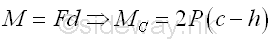
The free-body diagram of the entire beam is
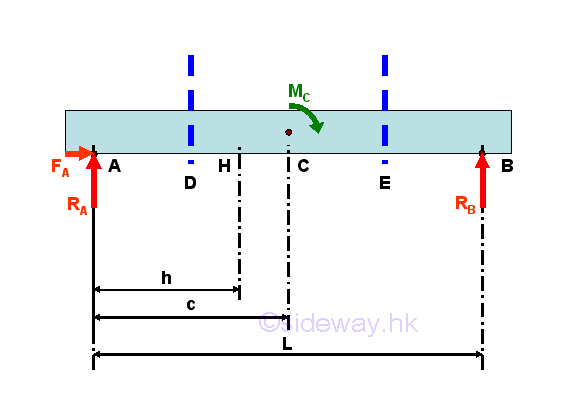
The reactions at the hinged and roller supports can be determined by the equilibrium equations.
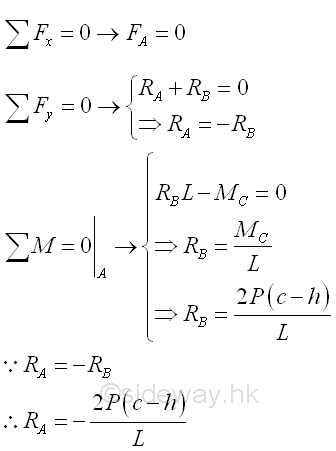
Internal forces can be determined by dividing the beam into two separated free body. Selecting a point D between A and C, i.e. A<D<C
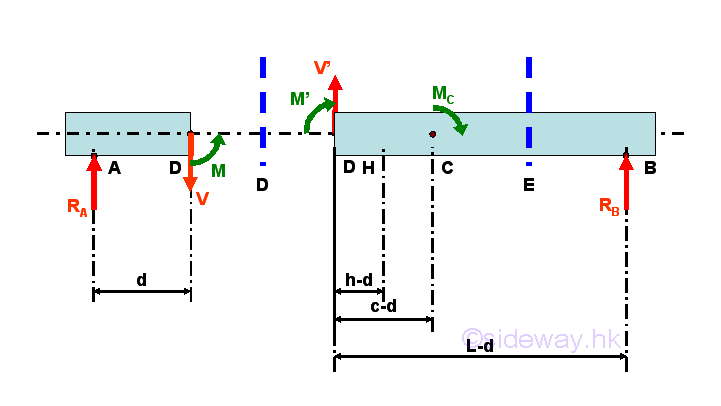
Consider the member section AD of length d, the internal forces at point D are
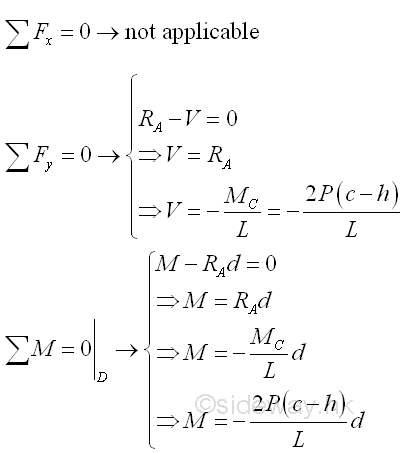
Point D is a random point between A and C. The shear force V is a constant and is equal to -2P(c-h)/L between point A and point C. And bending moment M is a linear function of point D. Therefore bending moment M=0 at point A and bending moment M=-2P(c-h)c/L at point C.
Selecting another point E between C and B, i.e. C<E<B
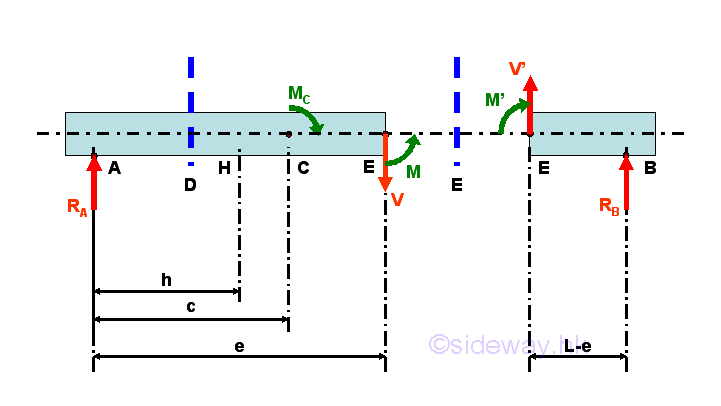
Consider the member section EB of length L-e, the internal forces at point E are
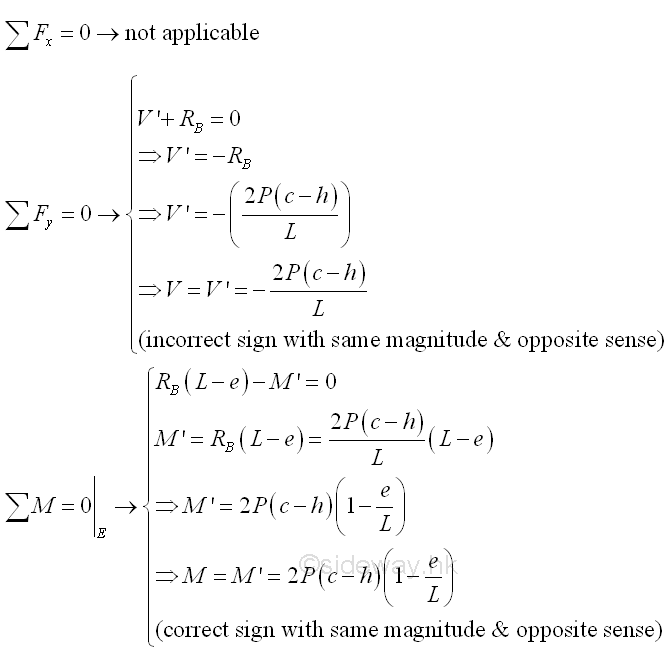
Point E is a random point between C and B. The shear force V is a constant and is equal to -(2P(c-h))/L between point C and point B. And bending moment M is a linear function of point E. Therefore bending moment M=2P(c-h)(1-c/L) at point C and bending moment M=0 at point B.
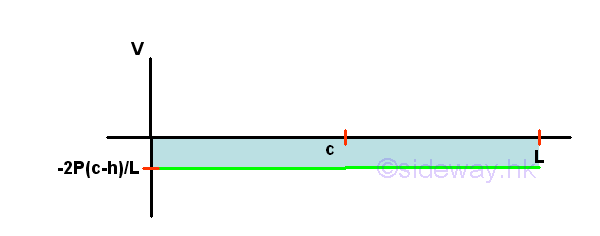
Shear Diagram
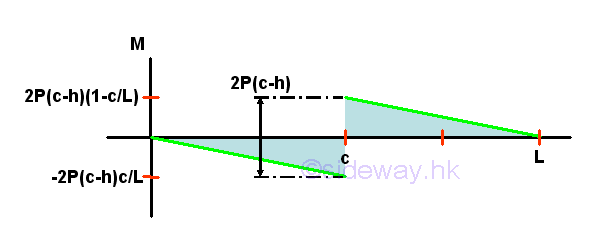
Bending Moment Diagram
©sideway
ID: 120800024 Last Updated: 8/29/2012 Revision: 0 Ref:
References
- I.C. Jong; B.G. rogers, 1991, Engineering Mechanics: Statics and Dynamics
- F.P. Beer; E.R. Johnston,Jr.; E.R. Eisenberg, 2004, Vector Mechanics for Engineers: Statics
Latest Updated Links
- Pentium(last updated On 7/3/2025)
- Intel CPU(last updated On 7/2/2025)
- Intel CPU History(last updated On 7/1/2025)
- FreeGLUT Windows Function(last updated On 1/27/2025)
- FreeGLUT Initialization Function(last updated On 1/26/2025)
- FreeGLUT(last updated On 1/25/2025)
- GLUT(last updated On 1/24/2025)
- OpenGL(last updated On 1/23/2025)
- XPower UC140 4-Port 140W PD3.1 GaN Travel Charger(last updated On 1/22/2025)
- XPower DX6 6 In 1 (2x3) 60W PD3.0 Sync & Charge Cable(last updated On 1/21/2025)
- XPower MF240 1.2M Magnetic Absorption 4 in 1 (2x2) Zinc Alloy 240W PD Sync & Charge Cable(last updated On 1/20/2025)

 Nu Html Checker
Nu Html Checker  53
53  na
na  na
na
Home 5
Business
Management
HBR 3
Information
Recreation
Hobbies 8
Culture
Chinese 1097
English 339
Travel 18
Reference 79
Computer
Hardware 257
Software
Application 213
Digitization 37
Latex 52
Manim 205
KB 1
Numeric 19
Programming
Web 289
Unicode 504
HTML 66
CSS 65
SVG 46
ASP.NET 270
OS 431
DeskTop 7
Python 72
Knowledge
Mathematics
Formulas 8
Set 1
Logic 1
Algebra 84
Number Theory 206
Trigonometry 31
Geometry 34
Calculus 67
Engineering
Tables 8
Mechanical
Rigid Bodies
Statics 92
Dynamics 37
Fluid 5
Control
Acoustics 19
Natural Sciences
Matter 1
Electric 27
Biology 1
